How do we plan for math with precision?

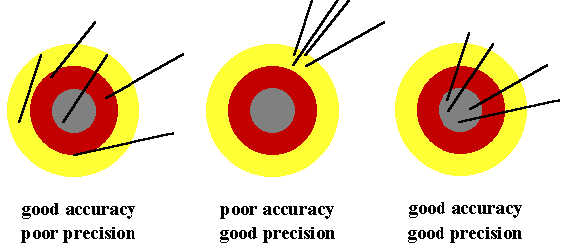
As the graphic below shows, when planning for math, we need accuracy and precision!

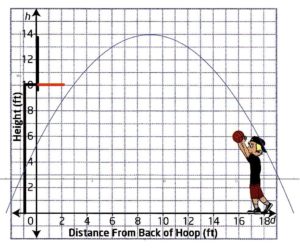
Let’s look at a real-world example of precision. March Madness is just around the corner. If teams want to make it to the finals, they must focus on precision. . The teams who want to win focus a lot of their time on the precision of their parabolas.

Planning for the perfect free throw

Parabolas, the elegant arched trajectory naturally formed by any projectile, determine the outcome of many ballistic sports. Parabolas have been studied extensively since people started throwing stuff at each other, but they reach their apex in basketball, where the field goals and free throws demand precision control of parabolas.

The Science of Parabolas

“But, it can’t be just any parabola. Success favors a fairly high arch. The ball must pass through the hoop with a little room to spare, and that limits the possibilities. The hoop is 18 inches in diameter, and the men’s ball is about 9.5 inches wide (women’s about 9.2). So if the men’s ball were thrown straight down from above — that is, at an angle of 90 degrees to the horizontal hoop rim, as in the classic Michael Jordan airborne dunk — there would be 4.25 inches of free space all around, a comfy margin.

But as the angle decreases and approaches the horizontal, the free space for a “nothing but net” shot gets much smaller. At 55 degrees, it’s about 2.5 inches. At 45 degrees, it’s down to 1.5 inches. And at 30 degrees, it’s basically impossible to get the ball straight into the basket, even with a full scholarship and more tattoos than a Hell’s Angels convention.”     -Washington Post Article, Tuesday, March 16, 2010

 Why am I thinking about precision, parabolas, and planning in January?

It’s simple. This time of year is when schools begin to look ahead and plan for state tests and end-of-course exams. With just few more months before these assessments, teachers must use precision in their instructional practice. I’m also thinking about precision because I’ll be out working with schools as we plan how to bring results and there’s a way to effectively plan.

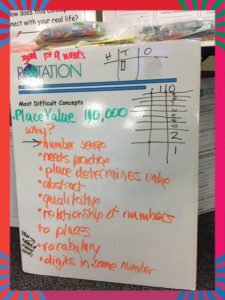
How do you know that it works? Here’s just one example. A few years ago, I worked with an Algebra I team to develop a precise plan for preparing their students to not just pass the exam, but to do so with much higher scores. There were 14 weeks remaining. We spent over three hours just determining which concepts were most important for the students to master before the test.

The teachers fully implemented the plan with amazing results. The school shot to #1 in their district in math and #10 in state. While seeing gains with all students, their lowest quartile had over 92% passing.

This method of planning with precision has been repeated hundreds of times since then and it works every time. Here’s how we planned.

Steps to Planning with Precision

1. Determine how many weeks and/or blocks of instruction remain before the final assessment.
2. Review all the concepts that will be tested and determine the most difficult concepts from the list.
3. List each of the most difficult concepts one at a time.
   

   Precision Planning for 3rd Grade

4. After listing each concept, determine why it is difficult. Be very specific about why the concepts are difficult for students to learn and/or teachers to teach.
   

   Precision Planning for Algebra I

5. Schedule these concepts to be taught in a teacher-guided, small-group setting to approximately ¼ of the class at a time while the rest of the class is working in pairs, teams, or independently. [In the Tabor Rotation Framework this is group is called Teacher Time and occurs each week at the elementary level and each unit at the secondary level.] 
6. Use the “whys” to guide your instruction, in these small groups, with precision. If time allows, “sprinkle” prerequisite skills, that might be missing, ahead of time.
7. Use as much hands-on and engaging instruction as possible when instructing these concepts.
8. Research how the concept is assessed on the state test and use this type of questioning with your small group of students.
9. Bridge from the activities and instruction used in the small group to the way it will be asked on the state test by using Exit Tickets, Exit Questions, Passports, and short quizzes.
10. If your precision is working, then celebrate and keep going!

As teachers in today’s schools, planning with precision can bring about much greater results—even if it impacts just one person—you!

For more about the value of guided math instruction, read the blog post, “The Extreme Value of Teacher-Guide Math in Teacher Time.”

If you want more specific steps for developing station activities that will prepare your students ALL YEAR LONG, read, “Powerful Math Stations.”

For you gutsy secondary-level teachers who want to jump in with both feet, take a look at the steps for “Getting Started with Algebra Math Stations.”